If you’re going out for pizza in Budapest, which would you choose to get you there; a smartphone with GPS or a drop of gel on a little maze? A team of scientists from Switzerland, Hungary, Japan and Scotland under the leadership of Empa, the Swiss Federal Laboratories for Materials Science and Technology, thinks that the gel might be your better bet because that little bit of plastic and goo is a chemical computer capable of navigating a maze faster than a satnav.
Navigation is essentially a matter of solving a maze in terms of how to get from point "A" to point "B." In GPS and other satnav systems, this usually involves brute computing power to try out the various paths and then pick the best one. This takes time and is dependent upon a written algorithm, which introduces a human element into the problem solving that may prejudice the answer.
The Empa team’s approach was to rely on what’s called "chemical computing." Contrary to popular belief, not all computers are electronic or even mechanical in nature. Some, for example, can use flowing water, and others use chemical reactions to solve complex problems through the laws of physical chemistry.
The Empa chemical computer is based on the Marangoni effect. In this, if a liquid exhibits a difference in surface tension, it will flow away from the area of low surface tension and toward the higher tension area. An example of this are the familiar "wine tears," where the water in a glass of wine pulls away from the lower tension alcohol, forming tears of alcohol on the sides of the glass that slide down.
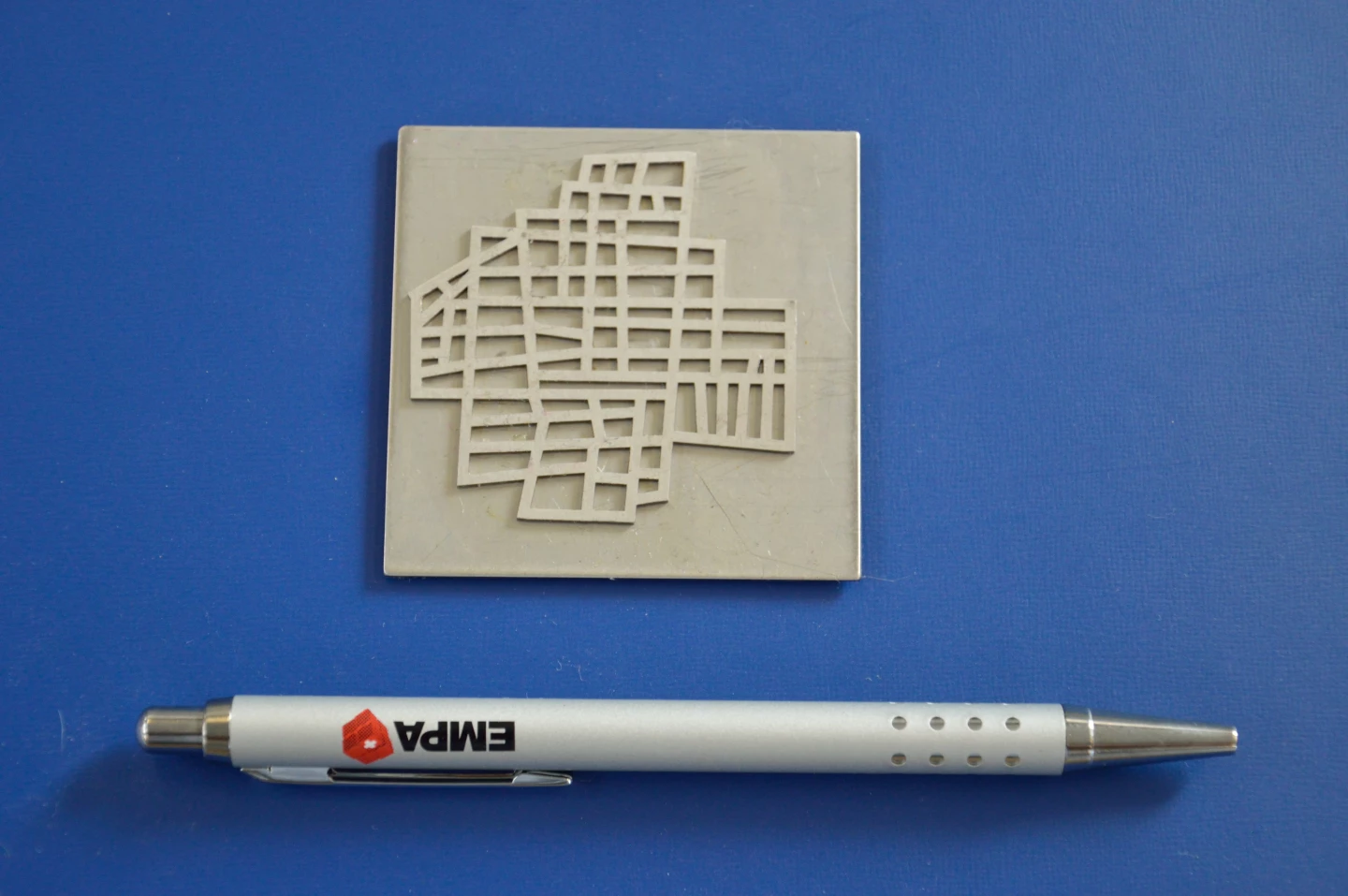
In the chemical computer, a maze is filled with an acidic gel with the highest concentration at the destination. Adding an alkaline solution mixed with dye at the starting point, this is drawn to the spot with the most acid by the differences in surface tension, with the dye leaving a trail behind.
As it does so, it follows the shortest path from start to finish because it is the one of the highest probability and therefore the least resistance. It may branch into other paths, but these are of a lower probability, so there isn't as much pressure to follow them, so the computer ignores them as water flowing downhill ignores unpromising side channels.
According to Empa, it is this that gives the process its speed. In one test maze based on the layout of Budapest measuring a few square centimeters, the chemical computer found the way to a local pizza shop faster than a satnav because the satnav had to look at all the alternatives and assess which one was the shortest, while the chemical computer went straight for the goal.
"The advantage of this chemical computer over its electronic counterpart is that it finds all the possible routes virtually in parallel," says Rita Tóth from Empa’s Laboratory for High Performance Ceramics. "A normal computer calculates step-by-step one possibility after another, which takes longer."
The Empa team sees the chemical computer as having application in transport system planning, logistics, experimental psychology, networks and graph theory, and robotics. It is currently working on adapting the method to larger and more complex maze problems.
The team’s results were published in Langmuir.
Source: Empa