It's often a case of swings and roundabouts. If you save money by buying a house out of town, you spend more time and money commuting. If you really measure the momentum of an electron, you have no idea where the little guy is located. And now, according to a new analysis by a pair of University of Texas electrical engineers, the better an object is hidden by an invisibility cloak at a given wavelength of light, the easier it is to see at other wavelengths. Swings and roundabouts.
The idea behind most types of invisibility cloaks is quite simple. Light rays are smoothly guided around objects, then brought back together so that they carry no sign that they were diverted around an object.
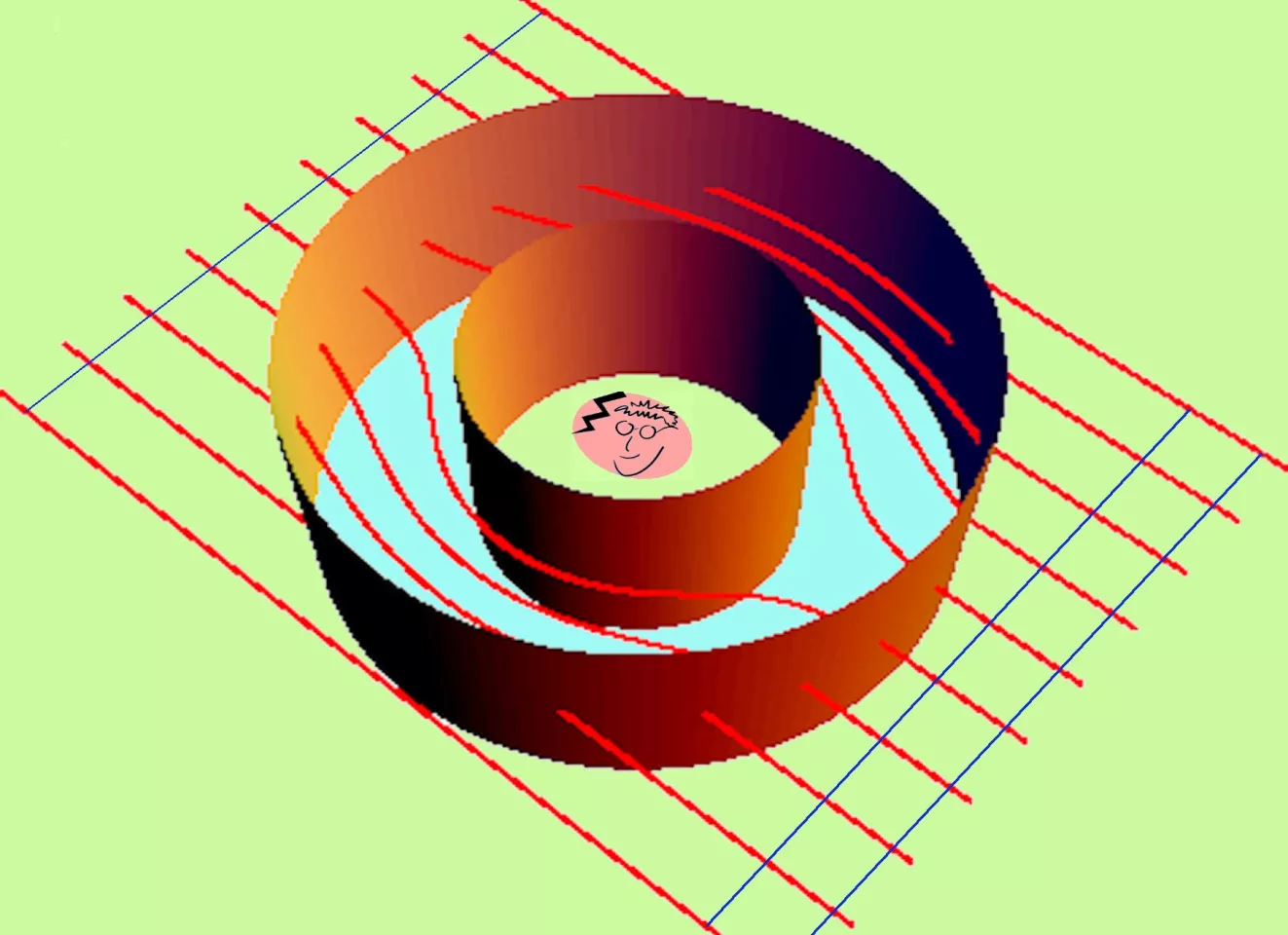
It is worth pointing out that, when this type of cloak is used, the person inside the cloak can't see the outside world as the light is bent around the central volume of the cloak, the cloakee receives no light from the world outside. Tiny peepholes can be inserted if needed, at a minor cost to the invisibility of the cloak.
However, the light that curves around the object travels further than does the light that passes just outside the material of the cloak. To make the phase of the light rays match when they are reassembled, this means that the wavefront velocity (thin blue lines in the picture above) in some portion of the material of the cloak must be greater than the speed of light (meaning the speed of light in a vacuum). No, hold onto your hats: relativity isn't violated, but we'll get to that in a moment.

In the picture above, the orange and purple lines indicate where the wavefronts of the light rays fall as the rays pass through the cloak. The red lines indicate the largest positive electric field intensity, and the blue lines the largest negative fields. Lime green indicates regions having little or no electric field.
Let's trace the light as it passes through the cloak. At the front of the cloak, a wavefront is slowed down so the light can bend around the center of the cloak. It remains attached to the parts of the same wavefront that pass outside the cloak (as seen on the upper and lower portions of the cloak.) The wavefront velocity in the front of the cloak is slower than light, just as would be true in an ordinary glass. At the rear of the cloak the pattern is quite similar, so the wavefront velocity there is also slower than light.
Here's the trick. For the cloak to be invisible, the wavefronts have to line up perfectly once they have passed through the cloak. Otherwise the light that was out of phase would cause interference and diffraction effects that would give away the cloak's location. But the figure above shows that the wavefronts within the cloak travel slower than light in a significant part of the cloak's thickness. The only way both of these can be true is if the wavefront velocity is faster than light in a region near the inner surface of the cloak – quite a lot faster than light.
As mentioned earlier, this doesn't violate special relativity, which actually states that no information can travel faster than the speed of light. To transmit information using a light beam, some characteristic of the light wave has to change as time passes. Time-dependent changes in the light wave that can carry information are called modulation. By varying the amplitude, phase, or frequency of the light wave or other electromagnetic radiation, we can enjoy over 200 channels of bad television in the comfort of our homes.
If you compare the red wavefront just entering the cloak on the left with the red wavefront just leaving the cloak on the right, they appear to be nearly identical. (They would be identical for a perfect cloak.) As the wavefront has not been changed by its passage through the cloak, no information has been transmitted, which means the bit of faster than light travel of the wavefronts internal to the material of the cloak doesn't violate relativity. There may appear to be holes in this illustration, but if you work it out in detail using Fourier analysis, there is no physically realizable situation in which relativity is violated.
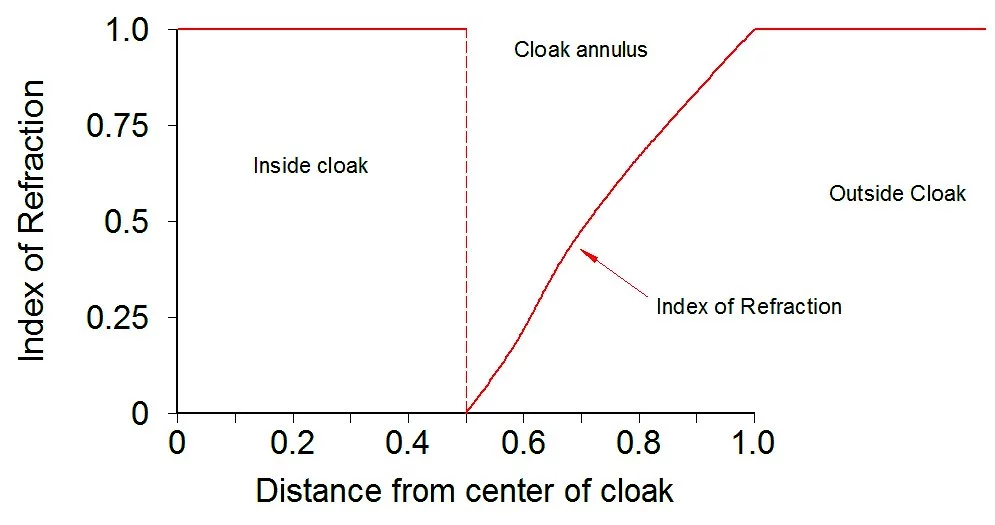
The ratio between the speed of light in a vacuum and the wavefront velocity in an optical medium is called the index of refraction n. As we need a region near the inner surface of a cloak in which the wavefront velocity is much greater than light, this means that the index of refraction must be less than one. The radial dependence for one of the early cloak designs appears in the figure above, and results in light of a particular frequency matching up nearly perfectly after passing through a cloak.
When you start to consider a range of colors of light, things get more complicated. Now the optical thickness of the cloak has to be equal to its diameter for all wavelengths. However, to have regions in which the index of refraction is less than one requires that the material of the cloak is dispersive, that is, that the index of refraction varies rapidly with the frequency of the light passing through. This makes it impossible to simply reproduce the index of refraction profile shown above, as that would imply that the index does not change with frequency, making it impossible for any region of the cloak to have an index smaller than one.
Indeed, it is not possible to make the optical and physical thickness of the cloak the same for rainbows of color. You can come close with light waves that have similar colors, but you can't actually get there. This implies that, as suggested in the lead photo, a cloak intended to hide an object from blue light is likely to exhibit a lot of scattered red light and interference effects that make the object stand out in red light, even while it is invisible in blue.
Clearly, there are a lot of details missing in this crude picture. Fortunately for us, University of Texas graduate student Francesco Monticone and his adviser, Prof. Andrea Alu of the Department of Electrical and Computer Engineering, have carried out a thorough analysis of broadband cloaking using today's most prevalent cloak designs. They find that "any cloak made of linear, passive, and non diamagnetic materials always increases the total scattering of the original uncloaked object. Suppressing the scattering in some frequency window has to be paid back, with interest, in the rest of the spectrum."
Monticone and Alu suggest that certain cloak designs having superconducting components may be able to provide partial broadband cloaking, but working this out will keep them busy for a while. Meanwhile, the date for purchase of a personal-sized invisibility cloak has probably been pushed out a decade or more. I am still hopeful about a successful development process, but then, you can't always get what you want.
Source: University of Texas via Arxiv