Mathematicians and engineers from MIT have used color-changing fibers to help develop a mathematical model that can predict the stability of a knot. By combining the theoretical with the empirical, the new approach can explain why one knot is better for a specific application than another.
People have been using knots for tens of thousands of years for such a wide variety of purposes that they could be said to tie civilization together. Even in the most advanced society, knots that would have been familiar to ancient Greek sailors are used on a daily basis for tying shoes, wrapping parcels, securing wires, making nets, or for simple decorations.
Yet, despite this ubiquity, knots aren't very well understood. Millennia of trial and error have produced a sound body of practical knowledge as to which knots are best for which tasks, but why a particular knot works well for one thing and not another and how to predict this has remained elusive.
According to MIT researchers, the main interest in knots from a scientific point of view has been mathematical, where knots have been regarded as a branch of topology, more concerned with theoretical patterns than physical bits of rope or twine.
"In mathematical knot theory, you throw everything out that’s related to mechanics," says Jörn Dunkel, associate professor of mathematics at MIT. "You don’t care about whether you have a stiff versus soft fiber – it’s the same knot from a mathematician’s point of view. But we wanted to see if we could add something to the mathematical modeling of knots that accounts for their mechanical properties, to be able to say why one knot is stronger than another."
In fact, two knots that look very similar to one another can have very different properties – as anyone who has tried to make a square knot and ended up with a granny can attest. This is why the MIT team turned to mathematical models.
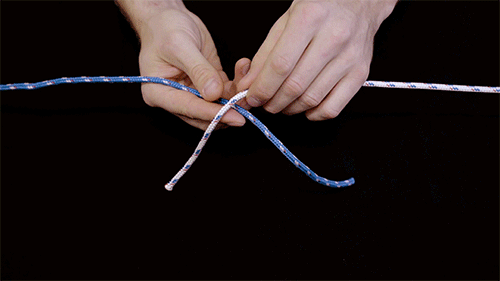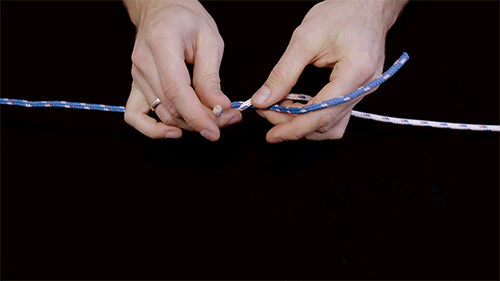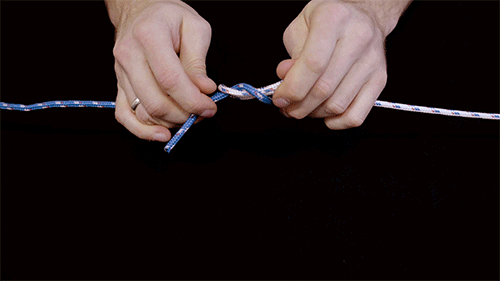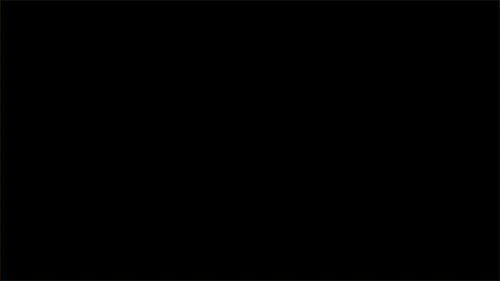
"These subtle differences between knots critically determine whether a knot is strong or not," says Dunkel. "With this model, you should be able to look at two knots that are almost identical, and be able to say which is the better one."
The model developed by Dunkel and PhD student Vishal Patil was based on the behavior of spaghetti and other rope-like structures, except that the strands were replaced in the simulation by small, discrete, spring-connected beads, the forces on which could be easily calculated.
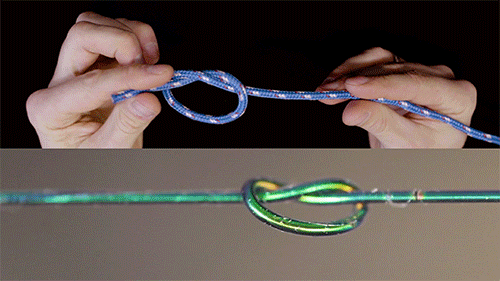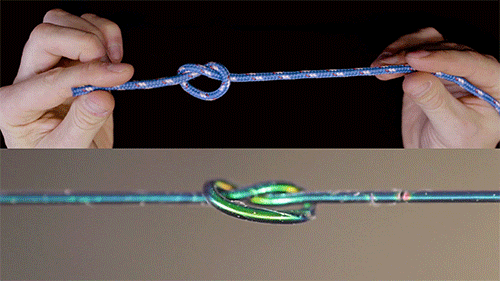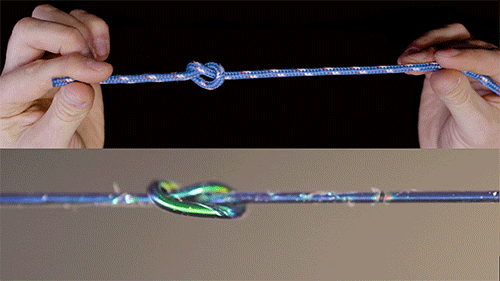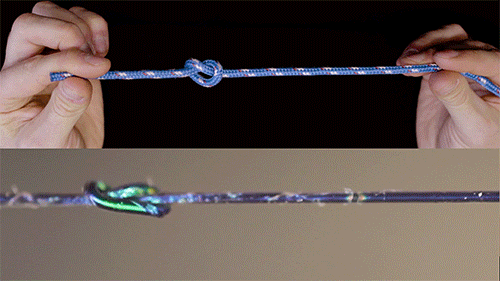
However, more was needed, so a color-changing fiber engineered by a group led by Mathias Kolle, the Rockwell International Career Development Associate Professor at MIT, was brought to bear. These fibers alter their color from one hue to another in response to stresses, providing visual clues to how a knot is performing.
By using the fibers to tie a variety of knots and photographing the results, Dunkel and Kolle were able to collect real-world data that could be fed into the model. This allowed them to sort out the knots according to their strength and stability.
By diagramming these knots, the team discovered rules that governed the knot's properties. They found that more times the strands of a knot crossed and the more times the direction of rotation changed, the stabler it is.
This means that if a strand rotates to the left and then to the right, the knot is stabler than if it rotates twice in the same direction. In addition, if two parallel strands loop against each other in the opposite direction, the knot is stronger.
"If you take a family of similar knots from which empirical knowledge singles one out as 'the best,' now we can say why it might deserve this distinction," says Kolle. "We can play knots against each other for uses in suturing, sailing, climbing, and construction. It’s wonderful."
The research was published in Science.
Source: MIT





